Hook: (8 minutes, slides 1-2)

Video: » Oracle Bone, Shang Dynasty  Students watch video and share noticings and wonderings about the video and oracle bones.

Relevance Explored: (10 minutes; slides 3-5):

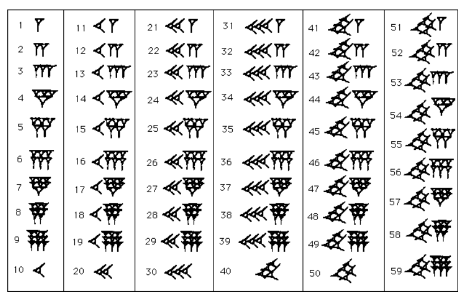
1) Worksheet: » Oracle Bone, Shang Dynasty Students examine the image of oracle bone numbers and try to write the number 3213 using oracle bone numerals.  

2) Then they try to write their own age and the current year.   

3) Students explore whether there was a “0” and how to write our current year without.  How does being “additive” take care of zero?  Would that always work?  

Mathematical Exploration: (10 minutes; slides 6-7)

1) Slides 6: Students watch a brief (4 minute) video about the history of Chinese mathematics.  Ancient Chinese Mathematics  

After writing a brief reflection on their worksheets (silently) have a quick whole class discussion about what was covered.  

2) Slide 7: Watch the video about using the numbers: Chinese Rod Numerals and students write at least 3 numbers with 3 or more digits and exchange and interpret with a partner.  

2) Slide 8: After reading https://mathshistory.st-andrews.ac.uk/HistTopics/Chinese_numerals/  Students write a short reflection or later and share with a partner.  

Student Discussion: (10 minutes; slide 9)

1. Watch the video about  Chinese Rod Numerals that discusses how rod numerals were used and are still used and currently taught in Chinese elementary schools (this video is sponsored by the Chinese government)    (Slide 6) (10 minutes)

Every Treasure Tells a Story: Counting Rods – A System of Numbers

2.  Closing work or Homework: (15 minutes if in class, Slide 9) Students read the summary page and write a reflection.  You can require the reflection to be as long or short as you wish.  Ideally students will share their reflections either by reading a partner’s and writing comments or by sharing their writing with their team and making a poster–possibly in the next class.  

Extension Activities: 

This continuation of counting rods exploration will take this further.  Students can either draw lines or you can provide rods (noodles, straws, popsicle sticks, etc. work well–or whatever “sticks” you might have–but each student or each pair need quite a few).  

• Another Hook: (5 or 12 minute video, 10 – 15 minutes including discussion. slide 10)
• Chinese Counting Rod Numerals — Multiplication or this shorter version by the same creators Counting Rod Multiplication)
• Students watch and then respond to “What do you think about the current use of counting rods? And How do you multiply by 10, 100, 1000, etc. 
• More Mathematical Exploration: (15 minutes; slide 9):  Chinese Counting Rod Numerals — Multiplication Students watch the video about multiplication and try using addition and subtraction with counting rods and then multiplication. 
• More Student Discussion: (10 minutes; slide 9) Short discussion:  Can counting rods be faster than calculators?  Is there anything about using counting rods that makes arithmetic more clear?