Hook: (10 minutes, slides 2-5)

There are two options for a hook to choose from.    Both options given students the opportunity to consider the meaning of base 10.  

• Option 1 (slide 3): This option is a more open ended option for students to try to represent what base 10 means to them using words, numbers or diagrams.  
• Option 2: (slide 4-5):  A more directed question will allow students to break down the number 4,392 and describe it using base 10.  Slide 5 will give students a guided breakdown and visual that will help them organize their ideas later in the lesson when considering base 60.

Mathematical Exploration: (30-45 minutes; slides 6-15)

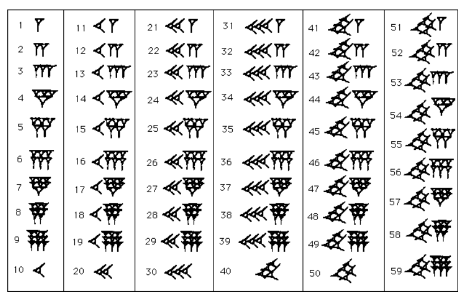
During the mathematical exploration students will learn about the Babylonian base 60 number system in cuneiform.  On slide 7 students will be given the Babylonian number system and ask them what base their number system was in.  The goal will be for students to recognize they have a base 60 model.  Students will have a class conversation about how it is difficult to conceptualize base 60 similar to how it is difficult to think in a new language you are just learning.  We often think in our native language rather than translate to the new language, this is similar to switching between mathematical bases.

On slide 8 students will read about how base 60 could have possibly been thought of in the Babylonian civilization and the possible connection back to time and the calendar or the hand model.  It is important to emphasize here that there is no clear answer in history as to why the Babyonlians used base 60, although nothing has been confirmed.  

Following this, on slide 9, students will complete a notice and wonder that shows the cuneiform symbols, transliteration numbers as well as decimal numbers.  The hope is that students would be able to discover that base 60 is about place value and exponential growth of base 60.  During this exploration it is essential that students are given the time to really try to problem solve and figure out the pattern.  It is important to give students a few minutes of independent thought time to process the information before they share out in groups.  If certain groups can figure out the first 2-3 examples, let them share with the whole class.  If students need extra support in how to organize their ideas slides 10-14 provide solutions.  

Once students have figured out how to convert numbers from cuneiform base 60 to decimal base 10 they can practice together.  It is recommended to allow students the chance to practice on whiteboards together following the Building Thinking Classrooms model.  Problems can be found on slide 15.  

Relevance Explored: (40-45 minutes; slides 16-18)

During relevance explored students will consider what life in Ancient Babylon was like.  Slide 17 leads them into an activity about imagining life without numbers through different Babylonian scenarios.  Provided are 4 different scenarios in which the Babylonians used mathematics: taxation, trade, construction and rationing.  In each of these scenarios students will be given a role to play in given scenarios, see handout for each scenario.  In each of these scenarios students will try to communicate without being able to use any numbers or vocabulary associated with numbers (ie. addition, subtraction, multiplication, etc).  The goal is for students to be able to acknowledge that without having a number system life the society would not have been able to function.  

Choose 2 groups to present their scenario in front of the class.  This is really important so that students have an example to specifically discuss with one another.  During debrief students will be able to discuss the efficiency and practicality of the students’ creative ways of communicating without numbers.  

Slide 18 sets students up to complete a Life in Babylon jigsaw share out.  Students will then be given the chance to research different aspects of the Ancient Babylonian lifestyle.   In small groups, students will explore one of the following areas in which Babylonians used mathematics in their civilization: taxes, architecture & construction, agriculture & livestock, rationing and trade.  Information and instructions that can be shared with students is found here.  Each student will complete a paragraph about their topic.  students have the freedom to add any type of information they want to their but it must be concise and not just paragraphs copy and pasted onto their document.  The links provided on their document are just intended to be a start for students to research.  They should be encouraged to also find additional resources based on information they read and find interesting.  The provided websites are intended to just be a start. 

Once students have completed research they will then do a jigsaw share out in their groups discussing what they have found.  They will give each student 2 minutes on a timer to share the information they learned about the use of mathematics.  It is helpful to make sure to display the time on the board for students to be able to pace themselves. It is recommended to let students know they cannot move onto the next person until after the 2 minutes is up.  If they feel they have finished sharing information, their group members should ask questions to fill in the rest of the 2 minute timer gap.

Student Discussion: (10-15 minutes; slides 19-20)

Students should be given the time to discuss and debrief the lesson together.   The discussion questions intended to let them reflect on Westernized perceptions of the development of Ancient cilivizations.  The questions provided are just suggested questions and should be edited and developed to meet the needs, identities and personalities of your students.  They can be considered in partners, small groups or whole class discussions based on your student population.

Extension Activities: (slides 21-24)

 If you would like to spend more time on than the lesson provided there are extension ideas ready to explore. Based the student population and task chosen additional research and development of content might be necessary:

• Extension 1: (slide 22) Birthday in Cuneiform – students can write their birthday in cuneiform base 60.
• Extension 2: (slide 23) Base 60 vs Base 10 – Students can read a NY times article, 60: Behind Every SEcond, Millennials of History which discusses the difference between base 10 and base 60.
• Extension 3: (slide 24)  Plimpton 322 – Students will learn about the ancient tablet Plimpton 322 by watching the youtube video Ancient Babylon Tablet – World’s First Trig Table.  Discussion questions are provided for student debrief.