Mathematical Topics: Does Society Need Math?
Mathematical Topics: Brazil
Suggested Age: 9th | 10th | 11th | 12th
Lesson Summary:
Through exploring research on societies that do not have number systems or awareness of numbers beyond 3, we consider the necessity of mathematics in our current society.
Remember that the lesson guides are just suggestions. Feel free to create your own options, use some of our suggested ones or even use it all. Most importantly consider your student population, involve them in the research and expand the lessons to fit your needs.
Our Lesson Plan
Our lessons are made to be printed or downloaded. Please do so through the link below.
Our Presentation
Our presentations are made to be printed or downloaded. Please do so through the link below.
Student Handouts
Our student handouts are made to follow the progression of the teacher guide. Download and edit to fit your students needs.
Hook: (15 minutes, slides 1-3)
Students will start by engaging in the ‘Rumors’ and Poster Activity. Pass out worksheet (The Piraha and Is Math Necessary). Students write their own response to “Was math invented or Discovered?” on an index card. Then walking around the read to another person and listen, then exchange cards. Next they share their “new” card with a different person until most information has circled. Then in small groups they organize the cards they have by “trends” or themes and finally groups share out their group poster information and students summarize their thoughts briefly on their worksheet.
Students will then engage in a small Group Discussion: (10 minutes, Slide #4)First have students examine the comic in slide 4 (created by Ben Rubenstein). Students talk with their “teams” and answer the two questions on their worksheet: What would life be like without numbers? And What is your own mathematical self-concept and where does it come from?
Relevance Explored: (20 minutes; slides 5-6)
Watch Video on slide #5 (video is 1 minute 18 seconds)) about Daniel Everett and the Piraha Tribe in South America and have students share their responses A Language without Numbers?
Then pass out Article 1 “How Humans Invented Numbers” (Option: This could be for HW and discussion next class). How Humans Invented Numbers—And How Numbers Reshaped Our World and have students discuss in their groups.
Mathematical Exploration: (15 minutes; slide 7)
Half of the students in each small group get one of the two articles, “When did Humans First Learn to Count” When did humans first learn to count or “The Birth of Numbers.” : The Birth of Numbers
Students then answer the questions carefully on their own worksheet. Proceed to “Spread the Knowledge” by sharing what they learned from their article, listening to information from the other article, and then creating a poster together to share what their team has learned. This information will all be useful for the socratic seminar discussion which can be either next or next class, depending on your timing.
Student Discussion: (20 minutes; slides 8-9)
Socratic Seminar (this might be the second day of class if you have 40 minute periods or the same day if you have extended classes). Have students sit in a circle where everyone can see one another. Share all of the questions with students in advance so they can prepare. Encourage students that the discussion should be guided by them. Whenever they feel ready to move onto another question they can pose one that is shared or create a question of their own.
Extension Activities: (slides 36-41)
There is a lot of research about when we learn numbers and are numbers necessary for a society. Teachers could have students read more and do more research and then discuss more, depending on your class. Some references here:
- Extension 1: Everett, Caleb, Numbers and The Making of Us: Counting and the Course of Human Cultures, Harvard University Press, 2017.
- Extension 2: Sharing knowledge of the people who spent time there. (writings by Caleb Everett and Keren Madora (Caleb is the son of Keren and Daniel. Keren’s last name is now Madora.)
- Extension 3: Further readings
- Quantity Recognition Among Speakers of an Anumeric Language, by: Caleb Everett, Keren Madora https://onlinelibrary.wiley.com/doi/10.1111/j.1551-6709.2011.01209.x
- Numbers and the Making of Us, Everett, Caleb, Numbers and The Making of Us: Counting and the Course of Human Cultures, Harvard University Press, 2017. (Caleb is the son of Daniel Everett and Keren Madora) https://www.hup.harvard.edu/catalog.php?isbn=9780674504431
- Brief Khan Academy description of counting and The Piraha: https://www.khanacademy.org/test-prep/mcat/social-sciences-practice/social-science-practice-tut/e/counting-systems-and-the-piraha-tribe-
- Books by Dan Everett: https://daneverettbooks.com/books/
- An Article comparing the Jarawara Tribe to the Piraha (The Jarawas (Jarawa: Aong, pronounced [əŋ][2]) are an indigenous people of the Andaman Islands in India. They live in parts of South Andaman and Middle Andaman Islands, and their present numbers are estimated at between 250–400 individuals. (from Wikipedia)) and finding the Jarawara Tribe to have a somewhat more numerical fluency: “Numerical cognition among speakers of the Jarawara language: A pilot study and methodological implication,” by Caleb Everett, May, 01, 2012, Proceedings of the 35th Annual Penn Linguistics Colloquium
chrome-extension://efaidnbmnnnibpcajpcglclefindmkaj/https://repository.upenn.edu/server/api/core/bitstreams/d475e037-aee5-41dc-9ea2-6c39f0c8c491/content
More Lessons
Below you’ll find our resources which are filterable by their age group and mathematical topic.

Kaktovik Numerals & Base 20

Tessellations in West African Hair Braiding

Density in Dominican Cakes compared to American Cakes

Hypatia of Alexandria & Conic Sections
Ancient Egyptian Base 10 Number System & Doubling Method

Maya Calendar & Base 20 Number System
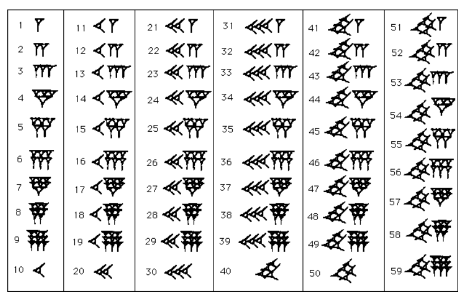
Ancient Babylon Base 60 Number System

The Origins of Fibonacci’s Sequence

African Baskets & Regular Polygon Rotations

Ancient Mathematical Puzzles: Magic Squares and Hexagonal Tortoises

Oracle Bones & Counting Rods

