Hook: (5 minutes, slides 2-3)

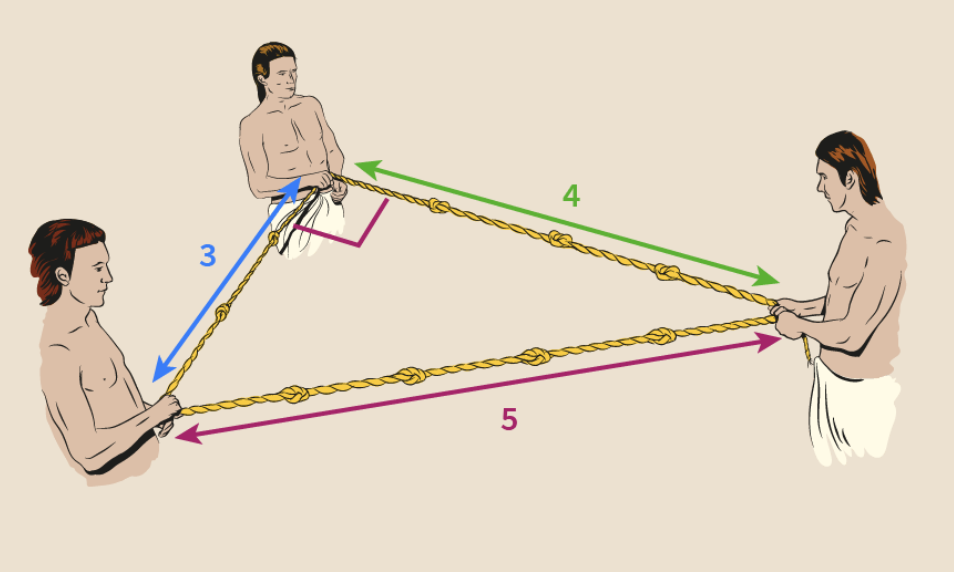
Before you pass out the worksheet have students carefully examine the two images on slide #2..  Then students share what they think may be relevant about the images.  Students will then look at the images with numbers (slide 3) as you pass out the worksheet and ask again what they notice and what they wonder.  Students should also write their ideas/questions on the worksheet. 

Relevance Explored: (15 minutes; slides 4-8):

1) Through the reading from “The Crest of the Peacock” on student worksheets and/or through the two videos listed, students learn more about the history of magic squares. 

2) Slides 5-7 explain some of the “magic” or connection to FengShui and how magic squares are still used in China and other parts of the world to aim for a balanced lifestyle.  

3) Students try to create their own magic squares of order 4.  

Student Discussion: (5 minutes)

Students discuss both the information about magic squares and their methods for creating magic squares.  

Hook: (5 minutes, slides 9-10)

Before you pass out the worksheet have students carefully examine the two images on slide #2..  Then students share what they think may be relevant about the images.  Students will then look at the images with numbers (slide 3) as you pass out the worksheet and ask again what they notice and what they wonder.  Students should also write their ideas/questions on the worksheet. 

Mathematical Exploration: (5 minutes; slides 12-13)

For the Hexagonal Tortoise problems, students can explore how to find the equations for different size “tortoises” (hexagons).  The worksheet lays out the shapes and asks for using each vertex in the grid that is given a number, from 1 to n (the number of vertices total), and to find the value of each hexagon is determined by adding up the 6 vertices at its points.  After examining  a “tortoise” that is fully labeled with numbers, students are asked to solve a 16 vertex  tortoise.  Then they are asked to find the formula for an “n” sided tortoise. 

Extension Activities: 

There are many other good lessons on magic squares, including this NCTM one with a good worksheet that focuses on Magic Squares in and how the ideas traveled around the world, with a focus on India, suggested for grades 6-8 but challenging enough for high school:

• https://www.nctm.org/Classroom-Resources/Illuminations/Lessons/Magic-Squares/
• And worksheet https://www.nctm.org/uploadedFiles/Content/Lessons/Resources/6-8/655-AS-Uncovering.pdf
• Or this one suggested for elementary through 5th grade: https://www.nctm.org/Classroom-Resources/Illuminations/Lessons/Magical-Magic-Squares/
• Or here is another brief fun worksheet: https://www.nctm.org/Classroom-Resources/Problems-of-the-Week/Magic-Square-Jumble/
• And a way to teach how to find all 3×3 magic squares: Magic Squares.docx
• A ‘Latin Square” (developed by Choi in Korea first!) example and exploration can be found here: https://nrich.maths.org/108/solution
• Or more about why hexagons are so important in nature:  https://www.zmescience.com/feature-post/natural-sciences/mathematics/hexagon-shape-nature-physics-13092021/